I used this problem once in a technical writing class I taught in 2000, but no students wanted (or could) tackle it. I still think it is valuable for students to write meaningfully about math and science, and I did not want the assignment to get lost. I doubt that I could reproduce the solution again. If anybody wants to use the assignment, please feel free. Here is the formal assignment as I typed it up in 2000.
This is the formal assignment for a max/min problem in engineering.
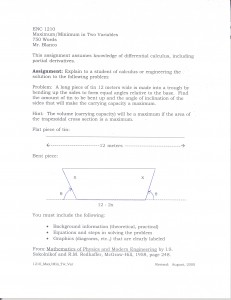
Formal Assignment: A long piece of tin 12 meters wide is made into a trough by bending up the sides to form equal angles relative to the base. Find the amount of tin to be bent up and the angle of inclination of the sides that will make the carrying capacity a maximum.
Hint: The volume (carrying capacity) of the trough will be maximized if the area of the trapezoidal cross section is a maximum.
This problem can be solved using trigonometry, basic geometry, algebra, and partial derivatives.
Below is the first page of the solution.
Solution, Page 1 of Max/Min Problem Using Partial Derivativ
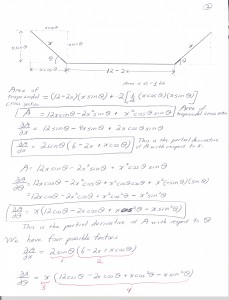
Below is page 2 of the solution. Notice that if we get a length x equal to 0 or an angle theta equal to 0, then we have to disregard those solutions because they are physically impossible or absurd.
Page 2, Solution. Max/Min Problem Using Partial Derivatives
Below is page 3 of the solution. The beauty of this problem is that we start with just a long piece of tin and we want to maximize the throughput (for example, the maximum amount of water that can go travel through the tin if we make it a trough) of the trough. That’s it. All we are given is the width of the material and a need to find the maximum throughput.
Page 3, Solution. Max/Min Problem Using Partial Derivatives